等效入口長度修正改善拉伸黏度預測 (Effective Entry Length Correction to Improve the Determination of Extensional Viscosity)

Revised: 2022/3/18

對於拉伸硬化的材料 (M1 material)，由 Fig. 4 可知，表觀入口黏度  η_ENT (apparent entrance viscosity) 受到 L/D 值的影響。

若以 L/D = 0 對應的 η_ENT 數據為例 (η_ENT = P₀/γ̇ _app)，透過 Cogswell、Binding、Gibson models 得到的拉伸黏度如 Fig. 6 所示。可以發現在低拉伸率下，Cogswell、Binding models 得到的拉伸黏度，明顯不符合牛頓流體 Trouton ratio 等於 3 的預期。 

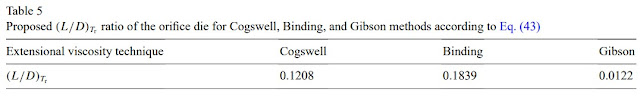
於是，Zatlukal et al. 提出等效入口長度修正的概念 (effective entry length correction)，改善上述三種 models 對低拉伸率下拉伸黏度的預測。以各個 models 在牛頓區間對 Trouton ratio 之預測均要回歸到 3 的概念，Zatlukal et al. 得到個別 model 理論上應對應的 L/D ratio (整理於 Table 5)。

這些特定 (L/D)_Tr 值所得到的拉伸黏度如 Fig. 8 所示。我們發現三種 models 在低拉伸率下，可以預測相同的拉伸黏度平台 (plateau)，且 Trouton ratio 等於 3。因此，相較於 Fig. 6，三種 models 對拉伸黏度的預測可以得到改善。

比較理論 (modified White-Metzner A model) 與模擬計算的拉伸黏度，我們可以由 Fig. 8 的比較結果得知，(i) 對於拉伸硬化的材料 (extensional hardening material, M1)，Cogswell model 高估在高拉伸率下的黏度，Binding model 高估低拉伸率下的黏度，而Gibson model 無法適當描述拉伸黏度的過衝 (overshoot)；(ii) 對於拉伸軟化的材料 (extensional softening material, M3)，三種模型得到類似的結果，它們的預測接近且斜率合理。

實驗室常用的商業化孔口模具為 L/D = 0.2 (orifice die)，因此，下方的 Figs. S1、S2 分別針對上述拉伸硬化 (M1) 及拉伸軟化材料 (M3)， 進行 L/D = 0.2 vs. (L/D)_Tr 之比較，藉以了解等效入口長度修正之效應。由於 Binding model 的理論 (L/D)_Tr 值 (= 0.1839) 相當接近實際模具的 L/D 值 (= 0.2)，故毋需進行長度的修正，然而，對於 Cogswell、Gibson models，長度的修正有其必要性。

Figure S1 拉伸硬化材料 (M1) 之 L/D = 0.2 vs. (L/D)_Tr。

Figure S2 拉伸軟化材料 (M3) 之 L/D = 0.2 vs. (L/D)_Tr。

Reference: M Zatlukal et al., "Improvement in techniques for the determination of extensional rheological data from entrance flows: Computational and experimental analysis," J. Non-Newtonian Fluid Mech. 107, 13 (2002).